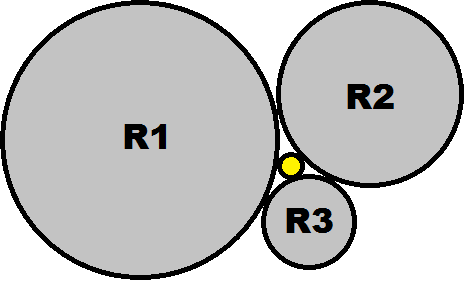
All circles in the picture above are tangent to each other. If radius $R1=2^{12}$, $R2=2^{11}$ and $R3=2^{10}$, calculate $r$, radius of the inner yellow circle. Let $x=\lfloor{r}\rfloor \times 10^{14}$.

We now define a new sequence as

$F(n) = F(n-1) + F(n-2) + (n-1)$ $\forall$ $n \geq 2$

with $F(0) = 0$ & $F(1) =1$.

Calculate sum $\{F(0) + F(1) + F(2) + ... + F(x-1) + F(x)\} \bmod {1000000007}$.