A regular hexagon with integer length $n$ units is divided in $6n^2$ equilateral triangles with sides of length 1 unit and this hexagonal lattice formed has a total of $3n^2 + 3n + 1$ lattice points.

For $n=3$, refer to the image below

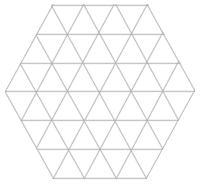
Let $H(n)$ denote the number of regular hexagons that can be formed by connecting any 6 points. Let $S(n)$ denote the summation of $H(k)$ for $1 \le k \le n$.

Given $H(3) = 36$ and $S(10) = 7942$, Calculate $S(10^{18})$.

Give your answer modulo $10^9+7$.



Hint: Can you observe any pattern?